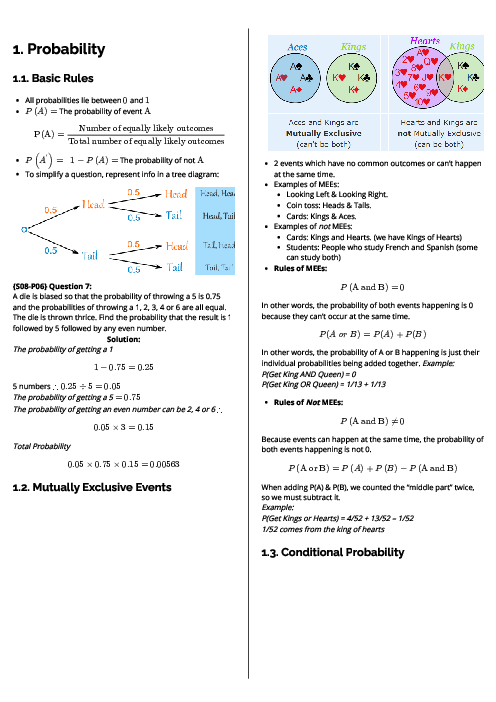
The complex number $u$ is defined by $u = \frac{{6 - 3i}}{{1 + 2i}}$.
a) Showing all your working, find the modulus of $u$ and show that the argument of $u$ is $ - \frac{1}{2}\pi $.
b) For complex numbers $z$ satisfying arg $\left( {z - u} \right) = \frac{1}{4}\pi $, find the least possible value of $\left| z \right|$.
c) For complex numbers $z$ satisfying $\left| {z - \left( {1 + i} \right)\,u\,} \right| = 1$, find the greatest possible value of $\left| z \right|$.
پاسخ تشریحی :
تحلیل ویدئویی تست
منتظریم اولین نفر تحلیلش کنه!