Sanket plays a game using a biased die which is twice as likely to land on an even number as on an odd number. The probabilities for the three even numbers are all equal and the probabilities for the three odd numbers are all equal.

a) Find the probability of throwing an odd number with this die.

Sanket throws the die once and calculates his score by the following method.

- If the number thrown is 3 or less he multiplies the number thrown by 3 and adds 1.

- If the number thrown is more than 3 he multiplies the number thrown by 2 and subtracts 4.

The random variable $X$ is Sanket’s score.

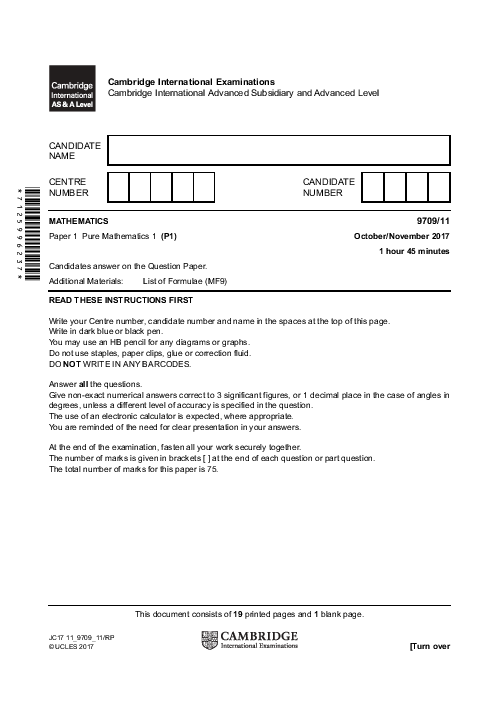
b) Show that $P\left( {X = 8} \right) = \frac{2}{9}$.

The table shows the probability distribution of $X$.

c) Given that $E\left( X \right) = \frac{{58}}{9}$, find $Var\left( X \right)$.

Sanket throws the die twice.

d) Find the probability that the total of the scores on the two throws is 16.

e) Given that the total of the scores on the two throws is 16, find the probability that the score on the first throw was 6.