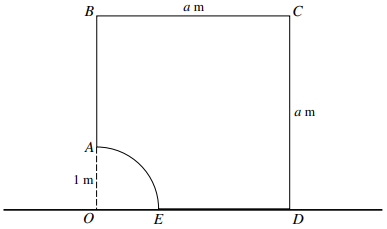
$ABCDE$ is the cross-section through the centre of mass of a uniform prism resting in equilibrium with $DE$ on a horizontal surface. The cross-section has the shape of a square $OBCD$ with sides of length $\alpha {\text{ m}}$, from which a quadrant $OAE$ of a circle of radius $1{\text{ m}}$ has been removed (see diagram).
a) Find the distance of the centre of mass of the prism from $O$, giving the answer in terms of $\alpha $, $\pi $ and $\surd 2$.
b) Hence show that
$3{\alpha ^2}\left( {2 - \alpha } \right) < \frac{3}{2}\pi - 2$,
nd verify that this inequality is satisfied by $\alpha = 1.68$ but not by $\alpha = 1.67$.
پاسخ تشریحی :
تحلیل ویدئویی تست
تحلیل ویدئویی برای این تست ثبت نشده است!
محتواهای آموزشی مشابه
فایل های پاورپوینت، ویدئو، صوتی، متنی و ...
پرسش و پاسخ های مشابه
سوال کنید یا به سوالات دیگران پاسخ دهید ...