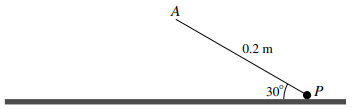
One end of a light inextensible string of length $0.2{\text{ }}m$ is attached to a fixed point $A$ which is above a smooth horizontal surface. A particle $P$ of mass $0.6{\text{ }}kg$ is attached to the other end of the string. $P$ moves in a circle on the surface with constant speed $vm{\text{ }}{s^{ - 1}}$, with the string taut and making an angle of ${30^ \circ }$ to the horizontal (see diagram).
a) Given that $v = 1.5$, calculate the magnitude of the force that the surface exerts on $P$.
b) Given instead that $P$ moves with its greatest possible speed while remaining in contact with the surface, find $v$.
پاسخ تشریحی :
تحلیل ویدئویی تست
تحلیل ویدئویی برای این تست ثبت نشده است!
محتواهای آموزشی مشابه
فایل های پاورپوینت، ویدئو، صوتی، متنی و ...
پرسش و پاسخ های مشابه
سوال کنید یا به سوالات دیگران پاسخ دهید ...