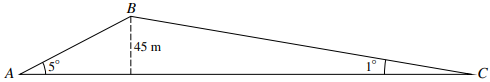
$AB$ and $BC$ are straight roads inclined at ${5^ \circ }$ to the horizontal and ${1^ \circ }$ to the horizontal respectively. $A$ and $C$ are at the same horizontal level and $B$ is $45{\text{ }}m$ above the level of $A$ and $C$ (see diagram, which is not to scale). A car of mass $1200{\text{ }}kg$ travels from $A$ to $C$ passing through $B$.

a) For the motion from $A$ to $B$, the speed of the car is constant and the work done against the resistance to motion is $360{\text{ }}kJ$. Find the work done by the car’s engine from $A$ to $B$.

The resistance to motion is constant throughout the whole journey.

b) For the motion from $B$ to $C$ the work done by the driving force is $1660{\text{ }}kJ$. Given that the speed of the car at $B$ is $15{\text{ }}m{\text{ }}{s^{ - 1}}$, show that its speed at $C$ is $29.9{\text{ }}m{\text{ }}{s^{ - 1}}$, correct to 3 significant figures.

c) The car’s driving force immediately after leaving $B$ is 1.5 times the driving force immediately before reaching $C$. Find, correct to 2 significant figures, the ratio of the power developed by the car’s engine immediately after leaving $B$ to the power developed immediately before reaching $C$.