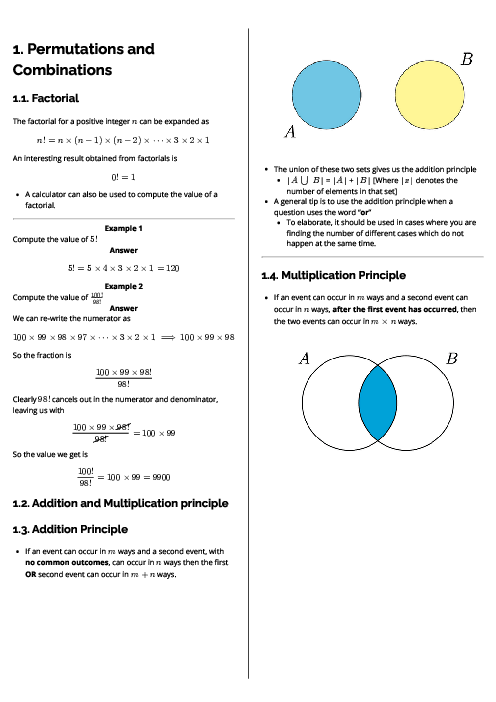
A particle $P$ is projected with speed $26{\text{ }}m{\text{ }}{s^{ - 1}}$ at an angle of ${30^ \circ }$ below the horizontal, from a point $O$ which is $80{\text{ }}m$ above horizontal ground.
a) Calculate the distance from $O$ of the particle $2.3{\text{ }}s$ after projection.
b) Find the horizontal distance travelled by $P$ before it reaches the ground.
c) Calculate the speed and direction of motion of $P$ immediately before it reaches the ground.
پاسخ تشریحی :
تحلیل ویدئویی تست
منتظریم اولین نفر تحلیلش کنه!