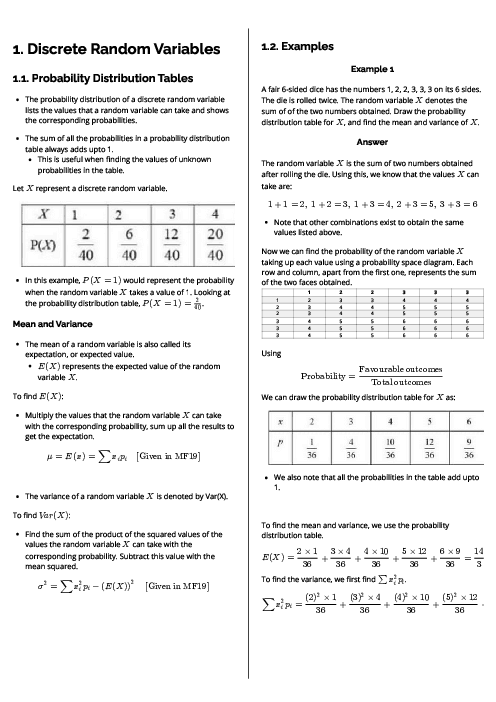
The weights of letters posted by a certain business are normally distributed with mean $20{\text{ }}g$. It is found that the weights of 94% of the letters are within $12{\text{ }}g$ of the mean.
a) Find the standard deviation of the weights of the letters.
b) Find the probability that a randomly chosen letter weighs more than $13{\text{ }}g$.
c) Find the probability that at least 2 of a random sample of 7 letters have weights which are more than $12{\text{ }}g$ above the mean.
پاسخ تشریحی :
تحلیل ویدئویی تست
تحلیل ویدئویی برای این تست ثبت نشده است!
محتواهای آموزشی مشابه
فایل های پاورپوینت، ویدئو، صوتی، متنی و ...
پرسش و پاسخ های مشابه
سوال کنید یا به سوالات دیگران پاسخ دهید ...