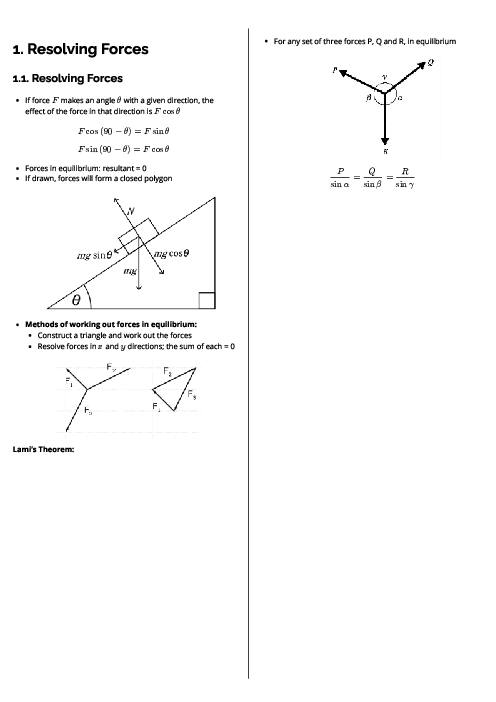
a) Express $4\sin \theta - 6\cos \theta $ in the form $R\sin \left( {\theta - \alpha } \right)$, where $R \gt 0$ and ${0^ \circ } \lt \alpha \lt {90^ \circ }$. Give the exact value of $R$ and the value of $\alpha $ correct to 2 decimal places.
b) Solve the equation $4\sin \theta - 6\cos \theta = 3$ for ${0^ \circ } \leqslant \alpha \leqslant {360^ \circ }$.
c) Find the greatest and least possible values of ${\left( {4\sin \theta - 6\cos \theta } \right)^2} + 8$ as $\theta $ varies.
پاسخ تشریحی :
تحلیل ویدئویی تست
تحلیل ویدئویی برای این تست ثبت نشده است!
محتواهای آموزشی مشابه
فایل های پاورپوینت، ویدئو، صوتی، متنی و ...
پرسش و پاسخ های مشابه
سوال کنید یا به سوالات دیگران پاسخ دهید ...