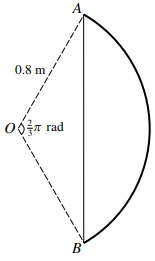
A bow consists of a uniform curved portion $AB$ of mass $1.4{\text{ }}kg$, and a uniform taut string of mass $m{\text{ }}kg$ which joins $A$ and $B$. The curved portion $AB$ is an arc of a circle centre $O$ and radius $0.8{\text{ }}m$. Angle $AOB$ is $\frac{2}{3}\pi $ radians (see diagram). The centre of mass of the bow (including the string) is $0.65{\text{ }}m$ from $O$. Calculate $m$.
پاسخ تشریحی :
تحلیل ویدئویی تست
تحلیل ویدئویی برای این تست ثبت نشده است!
محتواهای آموزشی مشابه
فایل های پاورپوینت، ویدئو، صوتی، متنی و ...
پرسش و پاسخ های مشابه
سوال کنید یا به سوالات دیگران پاسخ دهید ...