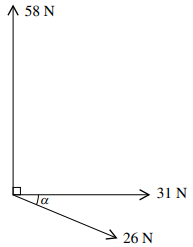
$X = 31 + 26\cos \alpha $, $Y = 58 - 26\sin \alpha $

$X = 55$, $Y = 48$

Resultant is $73N$ or

Direction is at ${41.1^ \circ }$ to i direction

Direction is at ${41.1^ \circ }$ to i direction or

Resultant is $73N$

Alternative solution for Q2

$\left[ {\tan {\theta _{12}} = 58/31,{\text{ }}{R_{12}}^2 = {{31}^2} + {{58}^2}} \right]$

${\theta _{12}} = {61.9^ \circ }$ and ${R_{12}} = 65.76$

$[Incl.{\text{ }}angle = {\left( {180 - {\theta _{12}} - \alpha } \right)^ \circ },$

${R^2} = {26^2} + {R_{12}}^2 - 2 \times 26{R_{12}}\cos \left( {incl.{\text{ }}angle} \right)]$

Incl. angle $ = {95.5^ \circ }$, Resultant is $73{\text{ }}N$

$\left[ {\sin \beta  = 26\sin 95.5/73;{\text{ }}\theta  = 61.9 - \beta } \right]$

Direction is at ${41.1^ \circ }$ to i direction