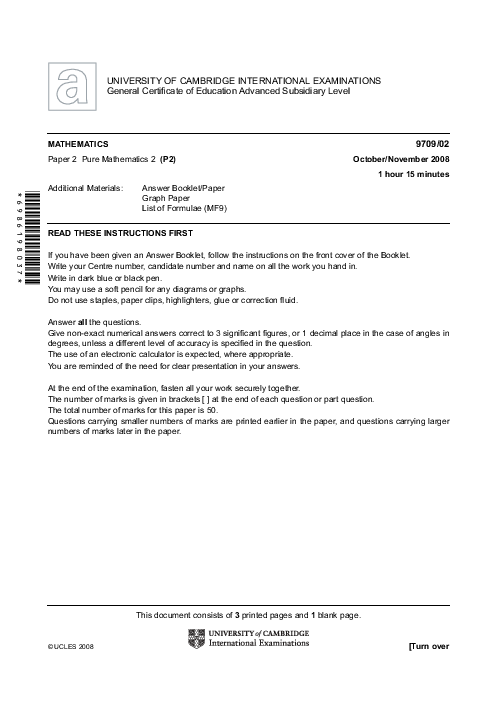
The diagram shows the velocity-time graph for a particle $P$ which travels on a straight line $AB$, where $vm{\text{ }}{s^{ - 1}}$ is the velocity of $P$ at time $t\,s$. The graph consists of five straight line segments. The particle starts from rest when $t = 0$ at a point $X$ on the line between $A$ and $B$ and moves towards $A$. The particle comes to rest at $A$ when $t = 2.5$.

a) Given that the distance $XA$ is $4{\text{ }}m$, find the greatest speed reached by $P$ during this stage of the motion.

In the second stage, $P$ starts from rest at $A$ when $t = 2.5$ and moves towards $B$. The distance $AB$ is $48{\text{ }}m$. The particle takes $12{\text{ }}s$ to travel from $A$ to $B$ and comes to rest at $B$. For the first $2{\text{ }}s$ of this stage $P$ accelerates at $3{\text{ }}m{\text{ }}{s^{ - 2}}$, reaching a velocity of $V{\text{ }}m{\text{ }}{s^{ - 1}}$. Find

b) the value of $V$,

c) the value of $t$ at which $P$ starts to decelerate during this stage,

d) the deceleration of $P$ immediately before it reaches $B$.