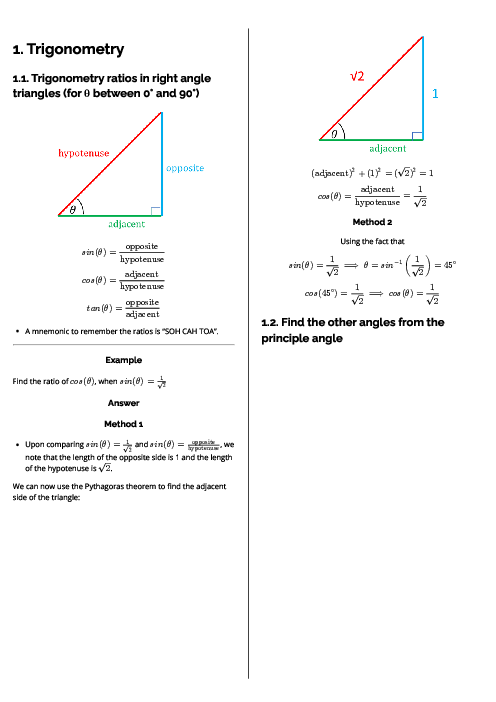
A particle $P$ is projected with speed $25{\text{ }}m{\text{ }}{s^{ - 1}}$ at an angle of ${45^ \circ }$ above the horizontal from a point $O$ on horizontal ground. At time $t\,s$ after projection the horizontal and vertically upward displacements of $P$ from $O$ are $x{\text{ }}m$ and $y{\text{ }}m$ respectively.
a) Express $x$ and $y$ in terms of $t$ and hence show that the equation of the path of $P$ is $y = x - 0.016{x^2}$.
b) Calculate the horizontal distance between the two positions at which $P$ is $2.4{\text{ }}m$ above the ground.
پاسخ تشریحی :
تحلیل ویدئویی تست
تحلیل ویدئویی برای این تست ثبت نشده است!
محتواهای آموزشی مشابه
فایل های پاورپوینت، ویدئو، صوتی، متنی و ...
پرسش و پاسخ های مشابه
سوال کنید یا به سوالات دیگران پاسخ دهید ...