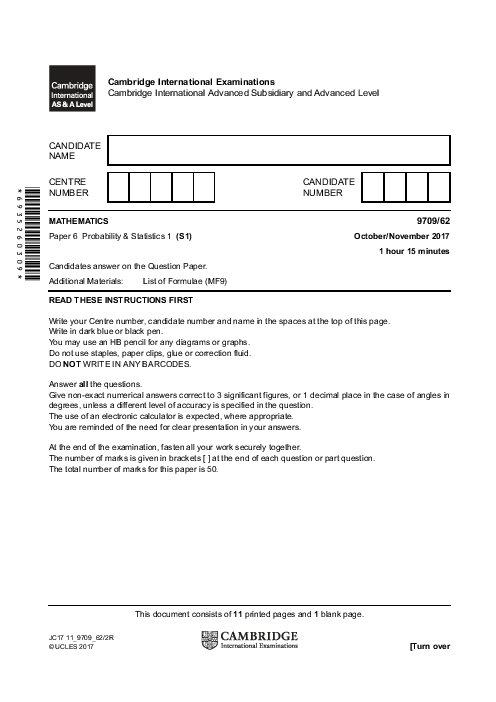
A light elastic string has natural length $2{\text{ }}m$ and modulus of elasticity $\lambda {\text{ }}N$. The ends of the string are attached to fixed points $A$ and $B$ which are at the same horizontal level and $2.4{\text{ }}m$ apart. A particle $P$ of mass $0.6{\text{ }}kg$ is attached to the mid-point of the string and hangs in equilibrium at a point $0.5{\text{ }}m$ below $AB$ (see diagram).
a) Show that $\lambda = 26$.
$P$ is projected vertically downwards from the equilibrium position, and comes to instantaneous rest at a point $0.9{\text{ }}m$ below $AB$.
b) Calculate the speed of projection of $P$.
پاسخ تشریحی :
تحلیل ویدئویی تست
تحلیل ویدئویی برای این تست ثبت نشده است!
محتواهای آموزشی مشابه
فایل های پاورپوینت، ویدئو، صوتی، متنی و ...
پرسش و پاسخ های مشابه
سوال کنید یا به سوالات دیگران پاسخ دهید ...