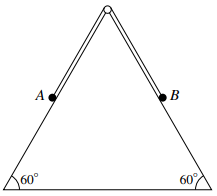
The diagram shows a vertical cross-section of a triangular prism which is fixed so that two of its faces are inclined at ${60^ \circ }$ to the horizontal. One of these faces is smooth and one is rough. Particles $A$ and $B$, of masses $0.36{\text{ }}kg$ and $0.24{\text{ }}kg$ respectively, are attached to the ends of a light inextensible string which passes over a small smooth pulley fixed at the highest point of the cross-section. $B$ is held at rest at a point of the cross-section on the rough face and $A$ hangs freely in contact with the smooth face (see diagram). $B$ is released and starts to move up the face with acceleration $0.25{\text{ }}m{\text{ }}{s^{ - 2}}$.

a) By considering the motion of $A$, show that the tension in the string is $3.03{\text{ }}N$, correct to 3 significant figures.

b) Find the coefficient of friction between $B$ and the rough face, correct to 2 significant figures.